More TAM fun

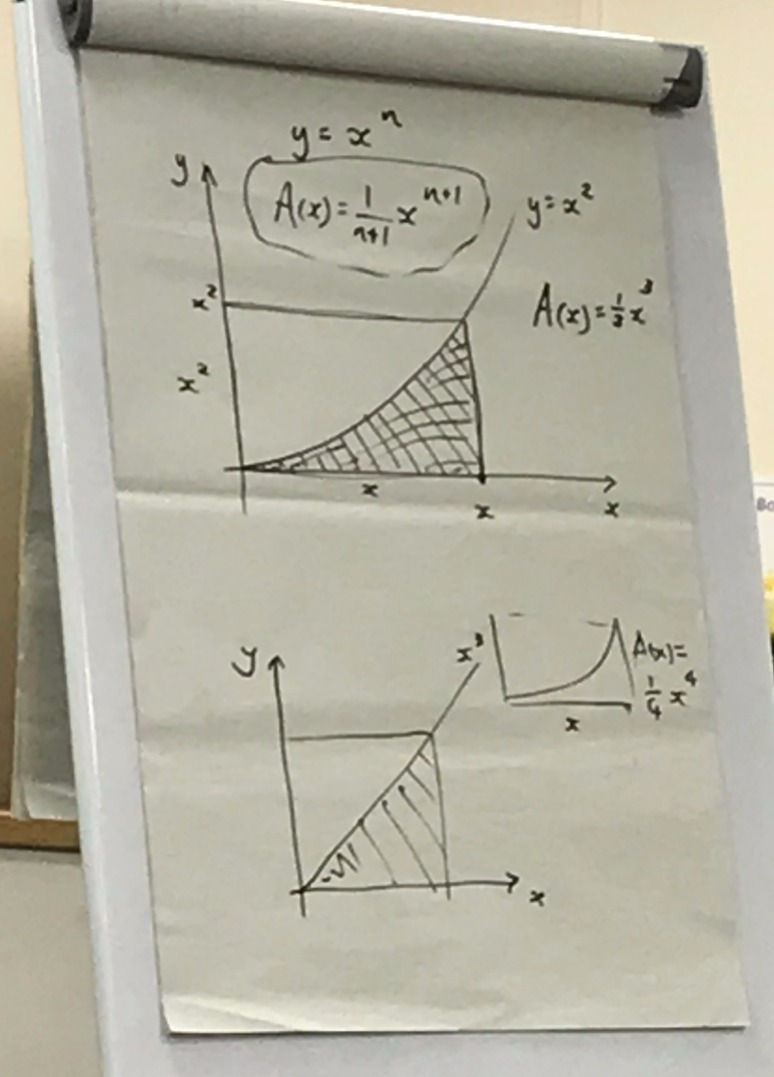
Every TAM experience I have makes me further question the way in which I teach A Level maths. I’m not saying all my lessons lower down the school are fun, but they’re definitely more engaging and interactive than what I usually do with year 12. In Birmingham earlier this week, I was stretched, challenged, questioned and questioned myself too, on approaches, teaching styles, course content and how best to support students to make progress. On Monday we focused largely on integration. We were asked to cut up graphs of y=x^2, y=x^3, y=x^4 and y=x^5 along the axes leaving only the positive quadrant, then along lines of our choosing that were parallel to the aces and met at the graph, to create a rectangle. Then came the scales! Yes, seriously. Thankfully it wasn’t just me that pulled a face at this, as I looked around the room to see other equally perplexed faces. All the y=x^2 rectangles were weighed together, then the y=x^3 rectangles, and so on. And their weights in grams recorded. Next we had to cut along the graphs, disposing of the piece above the graph and keeping the bit below? See where this is going? Then we weighed all the y=x^2 areas-under-the-graphs together, then the y=x^3 areas, etc. Again, the weights were recorded.

An underlying focus of the two days was conjecture and generalisation, so at this point in the task we were asked what we thought. Considering y=x with an area under the graph being half of the area of the rectangle, my group and I wondered if the area under the graph would be 1/(n+1), where n is the order of the graph. The results looked like this:

Now obviously, thinking about it afterwards, of course there would be this ratio relationship, because we know that integration is given by S y dx = x^(n+1)/n+1, but we had great fun getting there and now had a much stronger understanding of how integration works. I'm not sure if I'm going to look at this with my own class yet; I think it largely depends on how quickly I can get through the A Level content.

We looked at the Fundamental Theorem of Calculus afterwards, and it was pleasing to see how differentiating the area gives us the equation of the graph, and vice versa. The focus on Tuesday shifted towards polynomials and effective questioning in the classroom. Question styles discussed included scaffolding, funnelling and others, although the highlight for me was discussing with Matthew Man what his school use as a questioning structure. Following a question by the teacher and a response from the student, the teacher then asks another student to either Add to the response, Build on it, or Challenge it. This ABC approach sounds brilliant and I’m looking forward to using it as a technique to encourage discussion in my classroom. I have found that even after one day back in the classroom, my questioning has developed. I relished asking students to "convince me" all day! I really enjoyed exploring cubics and polynomials, and particularly appreciated the opportunity to practise dividing and factorising cubics using the grid method. I read about using the grid for this purpose on Resourceaholic a while ago, and today we looked at a factor table task from Integral that lent itself ever so nicely to be solved using a multitude of grids. Again, another fantastic task, although I was disappointed that I had to ask for a hint from the course leaders to complete it! We also discussed the links between factorised cubics, expanded equations, their graphs and table of values. It was interesting to analyse the connections.

The last thing we looked at over the two days was rich tasks: what it means for a task to be “rich” and how to create one. Interestingly, my view that rich tasks must be open-ended was challenged, and I now agree that a single point can be aimed for, as long as there are a variety of routes to get there. Most of the group also agreed rich tasks should be accessible yet challenging, should challenge understanding and should encourage discussion amongst students. We were introduced to RISPs (you need to look at these if you haven't seen them before!); I wish I knew about them sooner. Here’s my first attempt at a rich task. I used it today with my year 12 class and it brought out some brilliant discussion in a previously passive class. I plan to make a few more of these and add them to the website once I’m happy they meet the criteria above. Overall, I’ve taken in so much. I definitely need some time to process what I’ve learnt. I can’t emphasise enough how good the TAM course is. If you haven’t done it already, put it on your CPD list for next year - it’s so worth it.

#tam #mei #teaching #maths #alevel #integration #polynomials #cubic #richtask